Hostname: page-component-848d4c4894-hfldf Total loading time: 0 Render date: 2024-05-19T16:44:26.507Z Has data issue: false hasContentIssue false
  • English
  • Français

Weak approximation on the norm one torus

Part of: Exponential sums and character sums Arithmetic problems. Diophantine geometry Multiplicative number theory Linear algebraic groups and related topics

Published online by Cambridge University Press:  06 May 2024

P. Koymans Open the ORCID record for P. Koymans [Opens in a new window]  and
N. Rome Open the ORCID record for N. Rome [Opens in a new window]
P. Koymans
Affiliation:
Institute for Theoretical Studies, ETH Zurich, 8006 Zurich, Switzerland peter.koymans@eth-its.ethz.ch
N. Rome
Affiliation:
Graz University of Technology, Institute of Analysis and Number Theory, Kopernikusgasse 24/II, 8010 Graz, Austria rome@tugraz.at
Get access Rights & Permissions [Opens in a new window]

Abstract

For any abelian group 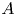$A$, we prove an asymptotic formula for the number of 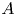$A$-extensions $K/\mathbb {Q}$ of bounded discriminant such that the associated norm one torus 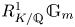$R_{K/\mathbb {Q}}^1 \mathbb {G}_m$ satisfies weak approximation. We are also able to produce new results on the Hasse norm principle and to provide new explicit values for the leading constant in some instances of Malle's conjecture.

Keywords

weak approximationnorm one torusHasse norm principleMalle's conjecturearithmetic statisticslarge sieve

MSC classification

Primary: 11N45: Asymptotic results on counting functions for algebraic and topological structures 14G05: Rational points 11L40: Estimates on character sums 20G30: Linear algebraic groups over global fields and their integers
Type
Research Article
Information
Compositio Mathematica , Volume 160 , Issue 6 , June 2024 , pp. 1304 - 1348
Check for updates
Copyright
© 2024 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Dedicated to Adèle Koymans-Funcken

References

Altuğ, S. A., Shankar, A., Varma, I. and Wilson, K. H., The number of $D_4$-fields ordered by conductor, J. Eur. Math. Soc. 23 (2021), 2733–2785.CrossRefGoogle Scholar
Bartels, H.-J., Zur Arithmetik von Diedergruppenerweiterungen, Math. Ann. 256 (1981), 465–473.CrossRefGoogle Scholar
Bhargava, M., The density of discriminants of quartic rings and fields, Ann. of Math. 162 (2005), 1031–1063.CrossRefGoogle Scholar
Bhargava, M., The density of discriminants of quintic rings and fields, Ann. of Math. 172 (2010), 1559–1591.CrossRefGoogle Scholar
Blomer, V., Goldmakher, L. and Louvel, B., $L$-functions with $n$-th-order twists, Int. Math. Res. Not. IMRN 2014 (2014), 1925–1955.CrossRefGoogle Scholar
Cohen, H., Diaz-y-Diaz, F. and Olivier, M., On the density of discriminants of cyclic extensions of prime degree, J. Reine Angew. Math. 550 (2002), 169–209.Google Scholar
Cohen, H., Diaz-y-Diaz, F. and Olivier, M., Counting discriminants of number fields, J. Théor. Nombres Bordeaux 18 (2006), 573–593.CrossRefGoogle Scholar
Darda, R. and Yasuda, T., The Batyrev-Manin conjecture for DM stacks, Preprint (2022), arXiv:2207.03645.Google Scholar
de la Bretèche, R., Kurlberg, P. and Shparlinski, I., On the number of products which form perfect powers and discriminants of multiquadratic extensions, Int. Math. Res. Not. IMRN 2021 (2021), 17140–17169.CrossRefGoogle Scholar
Ellenberg, J., Satriano, M. and Zureick-Brown, D., Heights on stacks and a generalized Batyrev–Manin–Malle conjecture, Forum Math. Sigma 11 (2023), Paper No. e14.CrossRefGoogle Scholar
Fouvry, E. and Klüners, J., On the 4-rank of class groups of quadratic number fields, Invent. Math. 167 (2007), 455–513.CrossRefGoogle Scholar
Frei, C., Loughran, D. and Newton, R., The Hasse norm principle for abelian extensions, Amer. J. Math. 140 (2018), 1639–1685.CrossRefGoogle Scholar
Frei, C., Loughran, D. and Newton, R., Number fields with prescribed norms, Comment. Math. Helv. 97 (2022), 133–181.CrossRefGoogle Scholar
Fritsch, R., Counting multi-quadratic number fields of bounded discriminant, Preprint (2019), arXiv:1902.03202.Google Scholar
Goldmakher, L. and Louvel, B., A quadratic large sieve inequality over number fields, Math. Proc. Cambridge Philos. Soc. 154 (2013), 193–212.CrossRefGoogle Scholar
Goldstein, L. J., A generalization of the Siegel–Walfisz theorem, Trans. Amer. Math. Soc. 149 (1970), 417–429.CrossRefGoogle Scholar
Granville, A. and Koukoulopoulos, D., Beyond the LSD method for the partial sums of multiplicative functions, Ramanujan J. 49 (2019), 287–319.CrossRefGoogle ScholarPubMed
Heath-Brown, D. R., A mean value estimate for real character sums, Acta Arith. 72 (1995), 235–275.CrossRefGoogle Scholar
Koukoulopoulos, D., The distribution of prime numbers, Graduate Studies in Mathematics, vol. 203 (American Mathematical Society, Providence, RI, 2019).CrossRefGoogle Scholar
Koymans, P. and Milovic, D. Z., On the 16-rank of class groups of $\mathbb {Q}(\sqrt {-2p})$ for primes $p \equiv 1 \bmod 4$, Int. Math. Res. Not. IMRN 2019 (2019), 7406–7427.CrossRefGoogle Scholar
Koymans, P. and Pagano, C., On Malle's conjecture for nilpotent groups, I, Trans. Amer. Math. Soc. Ser. B 10 (2023), 310–354.CrossRefGoogle Scholar
Koymans, P. and Rome, N., A note on the Hasse norm principle, Bull. Lond. Math. Soc., to appear. Preprint (2023), arXiv:2301.10136.Google Scholar
Macedo, A., Local-global principles for norms, PhD thesis, University of Reading (2021), https://drive.google.com/file/d/1hx4YUd_FjOxpoYqTxwo7iTKjr-TL6CGl/view.Google Scholar
Macedo, A. and Newton, R., Explicit methods for the Hasse norm principle and applications to $A_n$ and $S_n$ extensions, Math. Proc. Cambridge Philos. Soc. 172 (2022), 489–529.CrossRefGoogle Scholar
Mammo, B., On the density of discriminants of abelian extensions of a number field, Int. J. Number Theory 6 (2010), 1273–1291.CrossRefGoogle Scholar
Monnet, S., $S_4$-quartics with prescribed norms, Preprint (2022), arXiv:2210.06992.Google Scholar
Rome, N., The Hasse norm principle for biquadratic extensions, J. Théor. Nombres Bordeaux 30 (2018), 947–964.CrossRefGoogle Scholar
Sansuc, J.-J., Groupe de Brauer et arithmétique des groupes algébriques linéaires sur un corps de nombres, J. Reine Angew. Math. 327 (1981), 12–80.Google Scholar
Voskresenskiĭ, V. E., Birational properties of linear algebraic groups, Izv. Akad. Nauk SSSR Ser. Mat. 34 (1970), 3–19.Google Scholar
Voskresenskiĭ, V. E., Maximal tori without affect in semisimple algebraic groups, Mat. Zametki 44 (1988), 309–318; English transl. in Math. Notes 44 (1989), 651–655.Google Scholar
Wood, M. M., On the probabilities of local behaviors in abelian field extensions, Compos. Math. 146 (2010), 102–128.CrossRefGoogle Scholar
Wright, D. J., Distribution of discriminants of abelian extensions, Proc. London Math. Soc. (3) 58 (1989), 17–50.CrossRefGoogle Scholar