No CrossRef data available.
Article contents
On the primality of totally ordered q-factorization graphs
Part of:
Lie algebras and Lie superalgebras
Linear algebraic groups and related topics
Graph theory
Groups and algebras in quantum theory
Published online by Cambridge University Press: 20 March 2023
Abstract
We introduce the combinatorial notion of a q-factorization graph intended as a tool to study and express results related to the classification of prime simple modules for quantum affine algebras. These are directed graphs equipped with three decorations: a coloring and a weight map on vertices, and an exponent map on arrows (the exponent map can be seen as a weight map on arrows). Such graphs do not contain oriented cycles and, hence, the set of arrows induces a partial order on the set of vertices. In this first paper on the topic, beside setting the theoretical base of the concept, we establish several criteria for deciding whether or not a tensor product of two simple modules is a highest-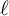
$\ell $-weight module and use such criteria to prove, for type A, that a simple module whose q-factorization graph has a totally ordered vertex set is prime.
Keywords
MSC classification
Primary:
17B10: Representations, algebraic theory (weights)
17B37: Quantum groups (quantized enveloping algebras) and related deformations
20G42: Quantum groups (quantized function algebras) and their representations
81R10: Infinite-dimensional groups and algebras motivated by physics, including Virasoro, Kac-Moody, $W$-algebras and other current algebras and their representations
05C20: Directed graphs (digraphs), tournaments
- Type
- Article
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press on behalf of The Canadian Mathematical Society
Footnotes
This work was developed as part of the Ph.D. project of the second author, which was supported by a PICME grant. The work of the first author was partially supported by CNPq grants 304261/2017-3 and 402449/2021-5, and Fapesp grant 2018/23690-6.
References
Barth, L. and Kus, D., Prime representations in the Hernandez–Leclerc category: classical decompositions. Preprint, 2020. arXiv:2012.15334Google Scholar
Brito, M. and Chari, V.,
Tensor products and q-characters of HL-modules and monoidal categorifications
. J. Éc. polytech. Math. 6(2019), 581–619.CrossRefGoogle Scholar
Brito, M., Chari, V., and Moura, A., Demazure modules of level two and prime representations of quantum affine
${\mathrm{sl}}_{n+1}$
. J. Inst. Math. Jussieu 17(2018), 75–105.CrossRefGoogle Scholar
${\mathrm{sl}}_{n+1}$
. J. Inst. Math. Jussieu 17(2018), 75–105.CrossRefGoogle ScholarChang, W., Duan, B., Fraser, C., and Li, J.,
Quantum affine algebras and Grassmannians
. Math. Z. 296(2020), 1539–1583.CrossRefGoogle Scholar
Chari, V.,
Integrable representations of affine Lie algebras
. Invent. Math. 85(1986), 317–335.CrossRefGoogle Scholar
Chari, V.,
Minimal affinizations of representations of quantum groups: The rank-2 case
. Publ. Res. Inst. Math. Sci. 31(1995), 873–911.CrossRefGoogle Scholar
Chari, V.,
Braid group actions and tensor products
. Int. Math. Res. Not. IMRN 2002(2002), 357–382.CrossRefGoogle Scholar
Chari, V., Davis, J., and Moruzzi, R. Jr, Generalized Demazure modules and prime representations in type
${D}_n$
. In: Representation theory, mathematical physics, and integrable systems, Progress in Mathematics, 340, Birkhäuser, Cham, 2021.Google Scholar
${D}_n$
. In: Representation theory, mathematical physics, and integrable systems, Progress in Mathematics, 340, Birkhäuser, Cham, 2021.Google ScholarChari, V., Moura, A., and Young, C.,
Prime representations from a homological perspective
. Math. Z. 274(2013), 613–645.CrossRefGoogle Scholar
Chari, V. and Pressley, A.,
New unitary representations of loop groups
. Math. Ann. 275(1986), 87–104.CrossRefGoogle Scholar
Chari, V. and Pressley, A.,
Quantum affine algebras
. Comm. Math. Phys. 142(1991), 261–283.CrossRefGoogle Scholar
Chari, V. and Pressley, A., A guide to quantum groups, Cambridge University Press, Cambridge, 1994.Google Scholar
Chari, V. and Pressley, A.,
Minimal affinizations of representations of quantum groups: The simply laced case
. J. Algebra 184(1996), 1–30.CrossRefGoogle Scholar
Chari, V. and Pressley, A.,
Factorization of representations of quantum affine algebras
. AMS/IP Stud. Adv. Math. 4(1997), 33–40.CrossRefGoogle Scholar
Duan, B., Li, J., and Luo, Y.,
Cluster algebras and snake modules
. J. Algebra 519(2019), 325–377.CrossRefGoogle Scholar
Etingof, P., Gelaki, S., Nikshychm, D., and Ostrik, V., Tensor categories, Mathematical Surveys and Monographs, 205, American Mathematical Society, Providence, RI, 2015.CrossRefGoogle Scholar
Frenkel, E. and Reshetikhin, N.,
The
$q$
-characters of representations of quantum affine algebras and deformations of
$\mathbf{\mathcal{W}}$
-algebras
. Contemp. Math. 248(1999), 163–205.CrossRefGoogle Scholar
$q$
-characters of representations of quantum affine algebras and deformations of
$\mathbf{\mathcal{W}}$
-algebras
. Contemp. Math. 248(1999), 163–205.CrossRefGoogle ScholarGurevich, M. and Mínguez, A.,
Cyclic representations of general linear p-adic groups
. J. Algebra 585(2021), 25–35.CrossRefGoogle Scholar
Hernandez, D.,
Cyclicity and
$R$
-matrices
, Selecta Math. (N.S.) 25(2019), 19.CrossRefGoogle Scholar
$R$
-matrices
, Selecta Math. (N.S.) 25(2019), 19.CrossRefGoogle ScholarHernandez, D. and Leclerc, B.,
Cluster algebras and quantum affine algebras
. Duke Math. J. 154(2010), 265–341.CrossRefGoogle Scholar
Hernandez, D. and Leclerc, B.,
Quantum affine algebras and cluster algebras
. In: J. Greenstein, D. Hernandez, K. C. Misra, and P. Senesi, (eds.), Interactions of quantum affine algebras with cluster algebras, current algebras and categorification. Progress in Mathematics, 337. Birkhäuser, Cham. doi: 10.1007/978-3-030-63849-8_2CrossRefGoogle Scholar
Hopkins, M. and Molev, A.,
A q-analogue of the centralizer construction and skew representations of the quantum affine algebra
. SIGMA 2(2006), Paper 092, 29 pages.Google Scholar
Jakelić, D. and Moura, A., Reducibility of tensor products of Kirillov–Reshetikhin modules from a duality perspective. In preparation.Google Scholar
Kang, S., Kashiwara, M., Kim, M., and Oh, S.,
Simplicity of heads and socles of tensor products
. Compos. Math. 151(2015), 377–396.CrossRefGoogle Scholar
Kashiwara, M., Kim, M., Oh, S., and Park, E., Monoidal categorification and quantum affine algebras II. Preprint, 2022. arXiv:2103.10067
Google Scholar
Moura, A., An introduction to finite-dimensional representations of classical and quantum affine algebras, Lecture Notes Published in Trabajos de matemática Série B 59, Publicaciones de la FaMAF - Universidad Nacional de Córdoba, Argentina, 2011.Google Scholar
Moura, A. and Silva, C., Three-vertex prime graphs and reality of trees. Commun. Algebra. doi: 10.1080/00927872.2023.2196345 arXiv:2204.10442CrossRefGoogle Scholar
Mukhin, E. and Young, C.,
Path description of type
$Bq$
-characters
. Adv. Math. 231(2012), no. 2, 1119–1150.CrossRefGoogle Scholar
$Bq$
-characters
. Adv. Math. 231(2012), no. 2, 1119–1150.CrossRefGoogle ScholarMukhin, E. and Young, C.,
Extended T-systems
. Selecta Math. (N.S.) 18(2012), 591–631.CrossRefGoogle Scholar
Nakajima, H.,
Quiver varieties and cluster algebras
. Kyoto J. Math. 51(2011), 71–126.CrossRefGoogle Scholar
Naoi, K., Equivalence via generalized quantum affine Schur–Weyl duality. Adv. Math. 389(2021), 107916. doi: 10.1016/j.aim.2021.107916 arXiv:2101.03573CrossRefGoogle Scholar
Oh, S. J. and Scrimschaw, T., Simplicity of tensor products of Kirillov-Reshetikhin modules: nonexceptional affine and
$G$
types. Preprint, 2020. arXiv:1910.10347
Google Scholar
$G$
types. Preprint, 2020. arXiv:1910.10347
Google ScholarQin, F.,
Triangular bases in quantum cluster algebras and monoidal categorification conjectures
. Duke Math. J. 166(2017), 2337–2442.CrossRefGoogle Scholar
Silva, C., A graph theoretical approach to prime simple modules over quantum loop algebras. Ph.D. thesis, Unicamp, 2022.Google Scholar